Optical telescope configurations provide the ability to magnify the apparent angular size of objects. The telescope is ubiquitous in optical setups and is also known as a beam expander “reducer”. It is heavily used in optics in various applications spanning from Microscopy to Beam shaping to Fourier optics.
The ratio and the distance between the focal lengths of those lenses or curved mirrors determine the angular magnification of the input beam. Common configurations include the Newtonian telescope configuration, Galileo telescope configuration, curved mirror based on axis configurations, and off-axis parabolic mirror-based telescopes.
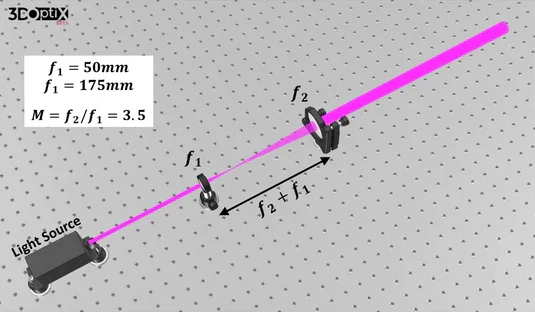
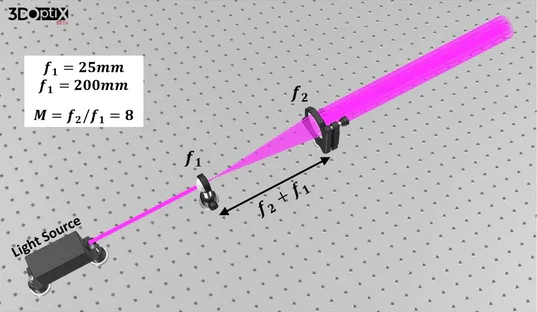
In this education webpage, the reader will experience the various parameters that control the accurate functionality of the telescope as well as the various telescope configurations. We will start with a simple Newtonian telescope, combined with two curved convex lenses, with focus distances of f1 and f2, which are associated with the first and the second lenses that the light hits.
In order to reach the right magnification M=f2/f1, the lenses should be separated by a distance of L=f2+f1. Any deviation from this distance will result in deviation from the accuracy of the output collimation.
In the following live simulation, the user can experience the sensitivity of those parameters. The user can change various lenses as well as can change the distance of the second lens from the first lens
* Select the mounts in order to change the optics
* Select the laser in order to change the wavelength
* Press the “Propagate Simulation” blue button to run a ray optic simulation
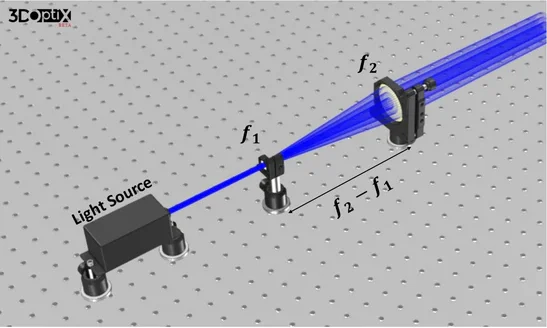
Next, we will explore the Galilean telescope configuration that is combined with a convex lens followed by a concave lens.
Try the 3DOptix online free Design & Simulation software
For more information and knowledge, one can register to 3DOptix free Design & Simulation online software at https://simulation.3doptix.com/
The software allows you to build any Optical design and simulate the optical path.

